Track Type

Track Type

SKY RAMP TECHNOLOGY

There are many options for inclined tracks. The U.S. Navy’s horizontal aerodynamic and ejection-seat test track at China Lake (below) has achieved Mach 4 sliding on steel rails. Note that high speed causes airflow under a sled and thus lift, so there is no friction from gravity as rail grips keep the sled from flying off the track. Wheeled sleds with Dunlop aluminum-wheeled jet-powered vehicles have achieved Mach 1. Magnetic Levitation (MagLev) now used for high-speed trains eliminates friction, but is very expensive to build and lacks the power for space launch. Iced rails or bobsled type tracks have not been used, however, frozen lakes have been used to achieve near Mach ground speeds. The track system may be of any type, monorail, dual track, multiple rails, slot and groove, or an iced track. The track can be built up a mountainside or inside a near vertical tunnel. The sled will fly through the air using any type of ground-mounted track to remain safely on course.

NASA's Maglev project conducted experiments to determine which type of Maglev track works best. Three types were tested and all worked. One track used a linear induction motor and no magnets on the sled, so it's a strong, durable unit, but it requires more power to create the magnetic field. The other two styles use a linear synchronous motor system with magnets right on the sled, so less electricity is needed to create the magnetic field. It uses less energy, but is a more complex system. The Boeing/NASA MagLev test track at Holloman Air Force Base (below) has achieved Mach 8 with small objects on its 10 mile horizontal test track. NASA would like to use Maglev to launch spacecraft as well, but the power needed to launch an RLV up a mountain is 100 times more than can now be stored and quickly released. As a result, MagLev propulsion is not presently and option for space launch.

The best angle for spacecraft launch is 70 to 80 degrees, but a as much as a 30 degree angle provides significant fuel savings. Ideally, this would be a straight track, but finding a mountain with a miles long steep incline is very difficult. The track could curve upward, but this induces G forces on passengers and places much greater stress on the track and RLV. Therefore, any curve must be near the beginning of the track well before the sled reaches 400 mph. Building a ramp up a mountainside may seem complex, but it's simple compared to the difficulties of building massive rollercoaster tracks.

Unless the sled is designed to go airborne and land at a nearby airstrip (or parachute down) for reuse after launching the RLV, some braking mechanism is needed to quickly stop the sled on the track after release of its payload. There are a dozen possible methods using simple existing technology, such as: curving the track, water braking (right), spoilers as air brakes, or hook and cables.

Horizontal Tracks

There is interest in using horizontal tracks for assisted launch since they can be built anywhere at lower costs. However, any velocity gained horizontally is lost as the craft must turn upwards through the Earth's atmosphere. This was confirmed in the 1980s by a detailed Boeing study of a horizontal space launch proposal code named SCIENCE DAWN. Boeing engineers concluded that horizontal takeoff requirement was an inappropriate application of rocket thrust-to-weight ratio, which was better applied to vertical takeoff.

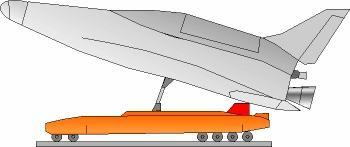A Soviet plan for a horizontal assisted spacecraft called the VKS-R (right) called for a wheeled sled to provide a Mach 0.5, zero altitude assist. Effective velocity gain compared to vertical launch would be 100 m/s. The sled would get the vehicle up to a velocity where the wings could provide lift, allowing lower-thrust engines to be used than in a vertical-takeoff design. This saved weight, but velocity losses during lifting flight to orbit almost cancel the advantage, resulting in the approach being unattractive in comparison to pure vertical-launch or air-launch designs.

Aerodynamic/physics expert, Dr. David Maker explains:

The horizontal launch drag calculation is admittedly messy, but one can get some rough estimates. (The drag coefficient is the primary source of the mess). Horizontal launch designers ignore the fact that the transition from horizontal to near vertical is not a short transition. For example, the centrifugal acceleration for mach 2 release in a 20km radius is ac~v^2/r= (2X340)^2/20,000~2g. Vectorially, add to that the orthogonal 0-2 g acceleration (add the gravity 1 g also) and you get conservatively about a 3g acceleration, probably near the limit the RLV can stand without structural reinforcement. So you need about 20km or more to get to near vertical. The second problem is that drag force since as a consequence you have to push against a lot of air at near sea level air densities.

Drag force Fd equals (1/2)(Cd)pAv^2 with the primary uncertainty here being Cd, given its somewhat quirky dependence on the Reynolds number, shape and lift drag. The problem (besides the ac=v^2/r problem) here is that (in their drag coefficient calculations) to get close to that m/mo~ .9 the RLV must be somewhat FAT&BLUNT the drag coefficient adjusted for transonic and supersonic effects and adding lift drag, Cd>1 (we will conservatively take Cd=1). Also because of the bluntness A~pir^2~3X2^2~10 for mac truck crossectional area (X-33 area also?), because this transition from horizontal to vertical is done near sea level air density p~1/2 for average up to about 6miles high, v~mach2~2X340m/sec, so v^2~4X10^5.

So Fd~ (1/2)(1/2)(1)4X10X10^5~2X10^5, L~20km=2X10^4. So approximate work FXL~ 2X10^5X(2X10^4)=2X10^10J. But the energy expended in the assisted launch is KE=1/2(mv^2). Here m~10^5 so KE due to boost (1/2)mv^2~.5X(10^5)X4X10^5~ 2X10^10 Joules. Thus the work to change the velocity from horizontal to near vertical is about the same as the energy expended to accomplish the assisted launch. Perhaps experts can whittle this down by using rough surfaces (like golf balls do), but conservative estimates were made here of Cd given the lift and supersonic drag coefficient effects.

While horizontal launch adds nothing to space launch, using rails to launch spaceplanes can save significant landing gear weight since it needn't be five times stronger to serve as take-off gear too, which is why Europeans plan to rail launch their Hopper sub-orbital spaceplane. Wings and engines can be much smaller as well. Nevertheless, if someone invests in a rail launch system for spacecraft, they should spend a bit more to build an elevated rail. A horizontal rail is also somewhat dangerous as a spacecraft will leave the rail just a few feet off the ground. Even a rail incline of 30 degrees can provide a significant assist to reduce the fuel required by any craft.